What's a watt?

You’ve likely heard of a watt – maybe you’ve replaced a 60 watt light bulb, or received your electricity bill and discovered that because you ran the AC too much last month, your household consumed 1200 killowatt-hours (900 kilowatt-hours is the average energy consumption of US households, per month).  Or maybe you’re into distance training and you hear people talk about “putting down some good watts.”  It’s difficult for me to conceptualize how much a watt is.  But I do know that a watt is related to energy consumption.  I also know eating is related to energy consumption.  And I know we measure food energy in calories.  Which takes me back to high school…

It was in my high school chemistry classroom that I first found myself excited by science.  And I’ll confess, one of the reasons… we got to burn stuff.  Seriously, we would set walnuts on fire.  Not just for fun, but for science!  We hear the lingo all the time… fat burning, carb burning, calorie burning.  It turns out, these metaphors are pretty much on the money.  When our bodies convert food to energy, the chemistry that happens is identical to burning, albeit more controlled.  Specifically, we break the carbon and hydrogen bonds in large molecules (like glucose), create new bonds with oxygen, and in the process get lots of energy out (as well as carbon dioxide, which we exhale, and water, which we, well, you know…).  

The "burning" of glucose creates energy (and carbon dioxide and water). 

In the case of metabolism, that energy allows our cells to go about their business keeping us alive.  In the case of fire, that energy is converted to light and heat: the flame.
 

So back to the burning walnuts.  In my chemistry class, we did a little experiment to measure the number of calories in a walnut.  We would do this by burning the walnut – quite literally – and capturing its heat output.  Check out the diagram below:

A simple experiment for measuring the caloric content of a nut.

This brings me to the actual definition of a food calorie: the amount of energy needed to raise the temperature of 1000 grams of water by one degree Celsius.  So to measure the number of calories in the walnut we would simply measure the temperature of a known amount of water before and after burning the walnut. 

 

In order to complete a half-ironman, I’m going to need a lot of energy, which means I’ll need to eat a lot of food (even during the race).  We are used to seeing energy content in food measured in calories.  

But there is an alternative way to measure energy stored in food – using joules.  Just as distance can be measured in either miles or kilometers, energy can be measured in calories or joules.  And as with distance measurements, where America seems to be the only place using miles, it would make things easier if we switched from calories to joules (you’ll see why in a bit).  There are 4,184 joules for each food calorie.  So that same Nutrition Label based on the units that nearly everyone else in the world uses would look like this:

Let me run with the distance analogy for a moment: Speed is a rate, measured in distance traveled per unit time (think: miles per hour, or meters per second).  Similarly, power – which is measured in watts – is a rate.  In this case, measured in joules per second.

This is why the joule is easier than the calorie to work with: it is very simply related to the watt.  

We’re finally set up to compare that 60 W light bulb to something a bit more tangible: the amount of energy we burn as humans, just by living.  Let’s do a quick calculation.  Let’s say you follow the USDA guidelines and consume 2000 calories per day.  To convert this to watts we have to run through some quick conversions – calories to joules (see, don’t you wish the USDA made its recommendations in joules?), and days to seconds.  That way we get an answer in joules per second, or watts.

 So you require just a bit more power than two standard 60 W light bulbs.

The human body consumes nearly 100 W of power when going about daily activities, like thinking at the office.  This is the equivalent power drawn by about 1.5 incandescent bulbs (60 W), or 7.5 compact fluorescent bulbs (13 W).

Now, to move that human body through the water, or to run or bike, you can guess that we NEED MORE POWER!

I can do a back-of-the-envelope estimate of how much power I must produce on the bike in order to hold a steady pace on a windless, flat road.  Remember Galileo and the two cyclists?  We learned that air resistance can be a big factor in cycling.  In fact, even on this windless road it is the primary force that I am working against.  To hold a constant speed, I must exert as much force to drive myself forward as the air resistance is pushing back against me with.  If I am going 20 mph… let me do some physics and math on the side here… I must produce 166 W of power.  I could power nearly 3 lightbulbs!  For comparison, elite cyclists can average 300 W of power, and can crank out more than 1,500 W in short bursts!

Hop on a bike and try to go 20 mph and you’ll need to produce an extra 166 W, on top of the 97 W your body already needs to perform its normal functions.

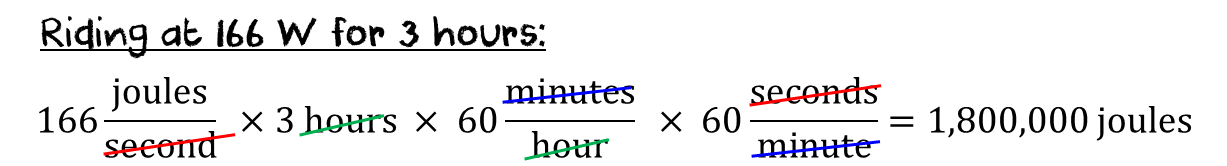
Now, let’s say I wanted to hold that pace for a 56 mi race (the bike leg of a half-ironman).  That can be easily divided by my 20 mph pace… it should take about 3 hours.  I just found that I have to produce 166 W of power to hold that speed.  So, my body will be using 166 joules every second for 3 hours.  

Sounds like a big number.  Let’s convert this to calories (since we are more familiar with that).  Remember that there are 4,184 joules per calorie, so we just divide our answer in joules by 4,184… I get 430 calories.  But here’s the kicker.  The human body isn’t a perfect food-to-mechanical energy conversion machine.  In fact, we are only about 20-25% efficient at converting food energy to motion.  So that means I will need 4 to 5 times that many calories in order to maintain that pace.  This is as much as 2150 calories!  Hence, this is what a typical grocery-store checkout looks like for me these days:

This brings me to my last point.  Since power is energy divided by time, we just saw that to calculate total energy consumed, we need to multiply power by time.  Or rearranging the equation we started off the day with:

 

This is why your electricity bill reports energy usage in kilowatt-hours.  It’s a weird unit.  I think we are used to saying things like “miles per hour” or “heart beats per minute.”  But in this case, we multiply your power use (in kilowatts) by the time of usage (in hours).  You can think of the hyphen in kilowatt-hours as a multiplication sign: kilowatt*hours.  Contrast this to miles per hour, where we think of “per” as the division sign.  Remember that watts are already in units of energy per time; so don’t read your energy bill as kilowatts per hour.  That would be the equivalent of saying “I drove my car at 60 miles per hour per hour.”  By instead multiplying wattage by time, we are left finally with energy.  So long story short, kilowatt-hours are a measure of energy, like joules, and no longer a rate, like watts. In fact, 1 kilowatt-hour is 3.6 million joules.

A 100 watt light bulb running for 10 hours consumes 1 kilowatt-hour of energy (100 watts * 10 hours = 1,000 watt-hours, or 1 kilowatt-hour).  Alternatively a 5000 W central air conditioner running for just 12 minutes also consumes a total energy of 1 killowatt-hour.  Below are some common appliances and gadgets, just to give you a sense of our energy-hungry lifestyles.  Remember that these are in watts: the rate at which these devices consume energy when they are on.  To find how much total energy they gobble up, you just multiply by their wattage by total running time.

Laptop	50 W
Microwave	1500 W
Clothes dryer	3400 W
Cell phone	1 W
Light bulb (incandescent)	60 W
Light bulb (compact fluorescent)	13 W
Central Air Conditioner	5000 W
Amateur Cyclist (like me)	100 – 200 W

So let’s take a look at that 1200 kilowatt-hour utility bill.  We can just multiply the power (1,200,000 watts) by one hour.  There are 3,600 seconds in an hour (60 minutes/hour * 60 seconds/minute).  This comes to be about 4 billion joules… that’s 4,000,000,000.  Or in calories: 1,032,505 – nearly one-and-a-half year’s worth of eating (on a 2,000 calorie diet)!